The Golden Angle

The golden angle is created by applying the golden ratio to the circumference of a circle. (The ratio of the two arcs defined by this angle is the same as the ratio between the larger arc and the full circle.) This angle is about 137.5 degrees.

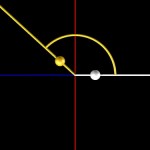
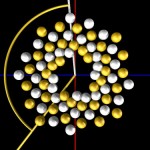
Positioning of many plants organ is directed by this angle as demonstrated below. First let us assume, we apply this angle for positioning balls with alternating colors and gradually increasing radius around the origin. Here comes the third ball in the arrangement shown above…

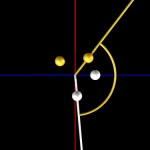
… this is the fourth ball then…

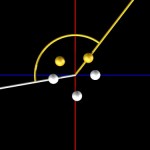
… this is the fifth ball…

… here comes the tenth ball…

Golden angle, 10 balls

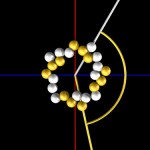
… here the 25^th …

… and here the 75^th.

Golden angle, 75 balls

Elements arranged this way can be organized into several spirals (Fermat’s spirals), with the numbers of such spirals being defined by Fibonacci numbers.

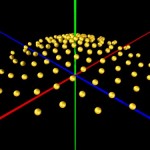
Here are some crude examples, how such a patterning can be used to model plant structures: First a cactus or a sunflower,…

Cactus-like distribution

… then a Christmas tree …

… and finally an ordinary shoot with the positions for ramifications.

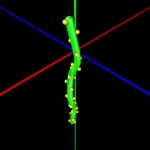
I became interested in the golden angle, because I was impressed by Aeonium plants I saw in Madeira and I wanted to model these plants. So here comes my approach: First I constructed the shortened shoot of such plants by applying the golden angle as indicated above.

Then I added branches to this shoot.

And finally the branches were used as the starting points of leaves. Some of the parameters of these leaves (inclination, size, form) are gradually changed in this model.

Aeonium plant

Further reading:

Applying the Golden Ratio to Web Layouts and Objects

SunFlower: the Fibonacci sequence, Golden Section

The Golden Ratio and the Quest for Beauty